x + 2 = x
For this purpose we will create task research force and will nose after her behavior. In a research group we will plug:
1) Child from preschool. He only begins to understand the elements of mathematics but already considers well.
2) Blonde, mother of child from preschool. Once at school taught mathematics and knew it well. Now she forgot everything.
3) Professor of mathematics De Colletage. Brilliantly owns a mathematical vehicle, decides the most intricate mathematical problems.
4) Nikolay Khyzhnjak. Engineer, addicts to mathematics, asserts that understands this abstract science.
You will say that it is not honest, to include itself in the complement of research group. But, respected, I thought of this group, I offered candidates in her composition, I assert final composition of research group. It is the most democratic principle. I honestly took advantage of democratic right to be select and won in the competition of candidates :)))
And so, we will ask our research group to decide a simple task and give the algebraic variant of her decision:
We have an unknown amount of hedgehogs to that added two grass-snakes. How many hedgehogs did turn out?
As a result a research group gave out next decisions.
1) Child from preschool. As I talked already, a child is clever and able to decide logical tasks, in that it is needed to guess the train of thoughts of author of task and give the same answer that waits from a child author of task. It is such method of training of future zombis, that is well able to guess that is waited from them by owners. A child answered the unknown amount of hedgehogs" will "Turn out. On a request to write down the decision of this task on the sheet of paper, a child answered that to write he is not yet able, but can draw a hedgehog.
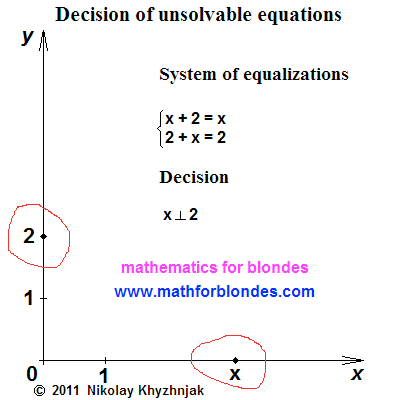
2) Blonde, mother of child from preschool. A blonde correctly decided a task after the row of leading questions. On a request to write down the decision through algebra, she began to reason: "Algebra, algebra... Nothing I remember... Algebra means - this unknown... Wow! We will designate the unknown amount of hedgehogs through "х". The amount of grass-snakes is known us and designating him is not needed, simply a number we write... Addition for us - it a sign "plus". Here"!. A blonde stretches out the sheet of paper, it is writtenin on that:
x + 2 = x
3) Professor of mathematics De Colletage. On a request to decide a task about hedgehogs and grass-snakes a famous professor was indignant, that it is ashamed to the great scientist to decide such child's tasks. He sent us after the decision of task in kindergarten. On our request to write down the algebraic decision of this task, a professor непонимающе looked at us, long thought and said: "Well, I will think above this problem". And was farther following...
For the algebraic reflection of decision of this task a professor worked out the special theory of three-cornered integrals, for that him was awarded with rank "academician". To the three-cornered integral the name of author was appropriated and he began to be named "Three-cornered integral De Colletage"...
For the decision of this concrete task the special research institute was created at the head with now already by an academician De Colletage. After a few years of the strained work, multivolume scientific work bore under the name "Practical application of three-cornered integral De Colletage in limits on the radius of cut on a dress"...
All of it nearscientific delirium we we will not study. We are interested only by a result. At once we open the last page of the last volume. There list of some organizations... We leaf pages to beginning of book in search of answer for a task... Somewhere in the middle of the last volume we find a line "Hired executed on account of working off scientific underbacks : .". Oho! This gang of easy riders from science on an even place managed to guzzle the whole lot of moneys! And before this line it is possible to find an answer for our task. "Х" is there written with plenty of reservations.
4) Nikolay Khyzhnjak. This witch-doctor of mathematics profoundly gave out a page under the name "Decision of unsolvable equalizations". According to his opinion, there is other task in that asked, how many hedgehogs will turn out, if to two hedgehogs to add the unknown amount of grass-snakes. He assures that both tasks have an identical decision :)))
By the way, there is another interesting task about grass-snakes and hedgehogs:
Mixture of grass-snake and hedgehog is a meter of the barbed wire. How mathematically to write down this expression?

Three-cornered integral De Colletage on the cut of dress not to apply :)))